Fundamental Theorem of Calculus

The fundamental theorem of calculus is a theorem that links the concept of integrating a function with that differentiating a function. The fundamental theorem of calculus justifies the procedure by computing the difference between the antiderivative at the upper and lower limits of the integration process. In this article, let us discuss the first, and the second fundamental theorem of calculus, and evaluating the definite integral using the theorems in detail.

Area Function

Lets consider a function f in x that is defined in the interval [a, b]. The integral of f(x) between the points a and b i.e. ∫baf(x)d(x),  is the area of that is bounded by the curve y = f(x) and the lines x = a, x =b and x – axis ∫xaf(x)dx.  depicts the area of the region shaded in brown where x is a point lying in the interval [a, b]. Assuming that the values taken by this function are non- negative, the following graph depicts f in x.

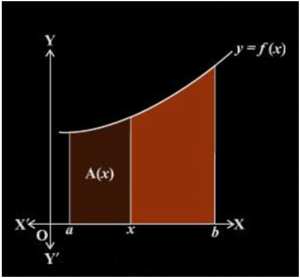
A(x) is known as the area function which is given as;

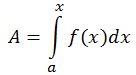
Depending upon this, the fundamental theorem of Calculus can be defined as two theorems as stated below:

First Fundamental Theorem of Integral Calculus (Part 1)

The first fundamental theorem of calculus states that, if the function “f” is continuous on the closed interval [a, b], and F is an indefinite integral of a function “f” on [a, b], then the first fundamental theorem of calculus is defined as:

F(b)- F(a) = a∫b f(x) dx

Here R.H.S. of the equation indicates integral of f(x) with respect to x.

f(x) is the integrand.

dx is the integrating agent.

‘a’ indicates the upper limit of the integral and ‘b’ indicates a lower limit of the integral.

The function of a definite integral has a unique value. The definite integral of a function can be described as a limit of a sum. If there is an anti-derivative F of the function in the interval [a, b], then the definite integral of the function is the difference between the values of F, i.e., F(b) – F(a).

Second Fundamental Theorem of Integral Calculus (Part 2)

The second fundamental theorem of calculus states that, if a function “f” is continuous on an open interval I and a is any point in I, and the function F is defined by

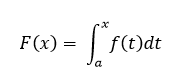
then F'(x) = f(x), at each point in I.

Here, the F'(x) is a derivative function of F(x).

How to Calculate Definite Integral?

Here are the steps for calculating ∫baf(x)dx

• Determine the indefinite integral of f(x) as F(x). It must be noticed that arbitrary constant is not considered while calculating definite integrals since it cancels out itself, i.e.,

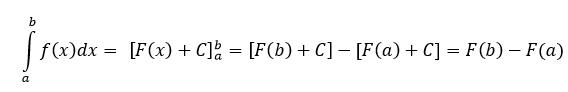
• Calculate F(b) – F(a) which gives us the value of the definite integral of f in x lying between the closed interval [a, b].